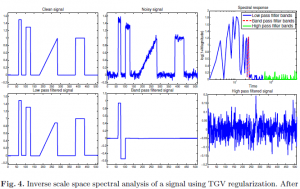
M. Burger, L. Eckardt, G. Gilboa, M. Moeller, “A spectral framework for one-homogeneous functionals”, Proc. Scale Space and Variational Methods in Computer Vision (SSVM), 2015.

Abstract:

This paper discusses a generalization of spectral representations related to convex one-homogeneous regularization functionals, e.g. total variation or $\ell^1$-norms. Those functionals serve as a substitute for a Hilbert space structure (and the related norm) in classical linear spectral transforms, e.g. Fourier and wavelet analysis. We discuss three meaningful definitions of spectral representations by scale space and variational methods and prove that (nonlinear) eigenfunctions of the regularization functionals are indeed atoms in the spectral representation. Moreover, we verify further useful properties related to orthogonality of the decomposition and the Parseval identity.

The spectral transform is motivated by total variation and further developed to higher order variants. Moreover, we show that the approach can recover Fourier analysis as a special case using an appropriate $\ell^1$-type functional and discuss a coupled sparsity example.

Tags: inverse scale space , one homogeneous , spectral TV , tgv

D. Horesh, G. Gilboa, “Multiscale orientation detection using the total variation transform”, Proc. Scale Space and Variational Methods in Computer Vision (SSVM), 2015.

Abstract:

Multi-level texture separation can considerably improve texture analysis, a significant component in many computer vision tasks.
This paper aims at obtaining precise local texture orientations of images in a multiscale manner, characterizing the main obvious ones as well as the very subtle ones.
We use the total variation spectral framework to decompose the image into its different textural scales. Gabor filter banks are then employed to detect prominent orientations within the multis-cale representation. A necessary condition for perfect texture separation is given, based on the spectral total-variation theory.
We show that using this method we can detect and differentiate a mixture of overlapping textures and obtain with high fidelity a multi-valued orientation representation of the image.

J.-F. Aujol, G. Gilboa, N. Papadakis, “Non local total variation spectral framework”, Proc. Scale Space and Variational Methods in Computer Vision (SSVM), 2015.

Abstract:

Eigenvalue analysis based on linear operators has been extensively used in signal and image processing to solve a variety of problems such as segmentation, dimensionality reduction and more.
Recently, nonlinear spectral approaches, based on the total variation functional have been proposed. In this context, functions for which the nonlinear eigenvalue problem $ \lambda u \in \partial J(u)$ admits solutions,

are studied.
When $u$ is the characteristic function of a set $A$, then it is called a calibrable set. If $\lambda >0$ is a solution of the above problem, then $1/\lambda$ can be interpreted as the scale of $A$.

However, this notion of scale remains local, and it may not be adapted for non-local features. For this we introduce in this paper the definition of non-local scale related to the non-local
total variation functional. In particular, we investigate sets that evolve with constant speed under the non-local total variation flow.
We prove that non-local calibrable sets have this property. We propose an onion peel construction to build such sets.
We eventually confirm our mathematical analysis with some simple numerical experiments.

Tags: calibrable sets , Non-local , nonlinear eigenvalue problem , scale , total variation

G. Gilboa, “A Total Variation Spectral Framework for Scale and Texture Analysis”, SIAM Journal on Imaging Sciences, Vol. 7, No. 4, pp. 1937–1961, 2014.

Abstract:

A new total variation (TV) spectral framework is presented. A TV transform is proposed which can be interpreted as a spectral domain, where elementary TV features, like disks, approach impulses. A reconstruction formula from the pectral to the spatial domain is given, allowing the design of new filters. The framework formulates a new representation of images which can enhance the understanding of scales in the $L^1$ sense and improve the analysis and processing of textures. An example of a texture processing application illustrates possible benefits of this new framework.

  Matlab Code

G. Gilboa, “Nonlinear Band-Pass Filtering Using the TV Transform”, Proc. European Signal Processing Conference (EUSIPCO-2014), Lisbon, pp. 1696 – 1700, 2014.

Abstract:

A distinct family of nonlinear filters is presented. It is based on a new formalism, defining a nonlinear transform based on the TV-functional. Scales in this sense are related to the size of the object and its contrast. Edges are very well preserved and selected scales of the object can be either selected, removed or enhanced. We compare the behavior of the filter to other filters based on Fourier and wavelets transforms and present its unique qualities.

  Matlab Code

Tags: nonlinear BPF , spectral TV , TV transform